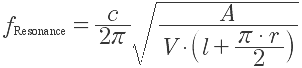Christian Ucke, Technical University Munich
cucke@ph.tum.de

Figure 1 was taken from an old German physics textbook¹ dating from 1906. So-called Helmholtz-resonators are fixed on a cross which can rotate easily on a needle bearing. With the right resonance frequency of the Helmholtz-resonators and enough acoustical power from a loudspeaker, this device starts to rotate anticlockwise (view from above).


Figure 1: Acoustical wheel from an old physics book.

Using the following formula, the resonance frequency of a Helmholtz-resonator can be calculated (figure 2, derivation in [2])



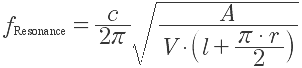
(V = volume of sphere; l = length of the cylinder; r = radius of the cylinder; A = area of the cylinder; c = velocity of sound)


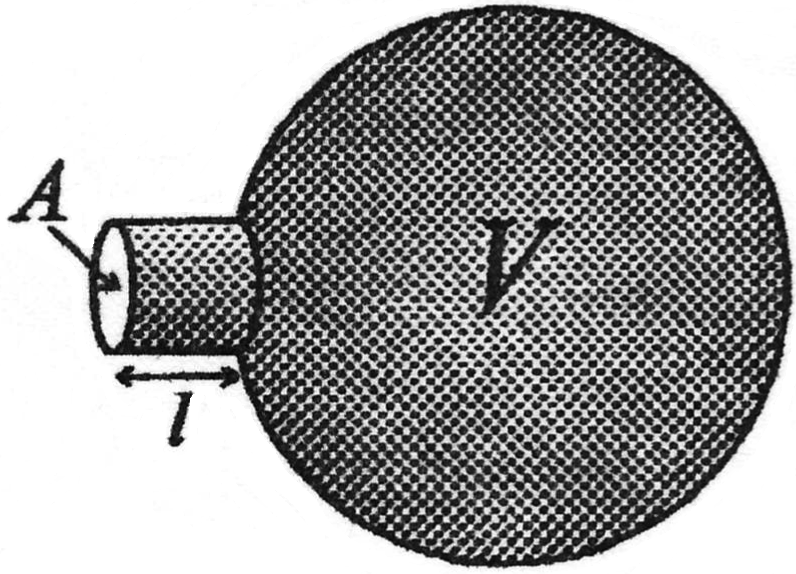Figure 2: Helmholtz Resonator.

The explanation of the rotation is very similar to that of the ell-known water-jet-boat (put-put-boat): the sine-stimulated loudspeaker causes a pressure variation inside the sphere at the resonant frequency. If the pressure in the sphere is smaller than outside, the air is sucked into the sphere from all directions (figure 3). If the pressure in the sphere is higher, the air is pressed out of the cylinder and has a preferred direction. Thus, a net force to the left side results.


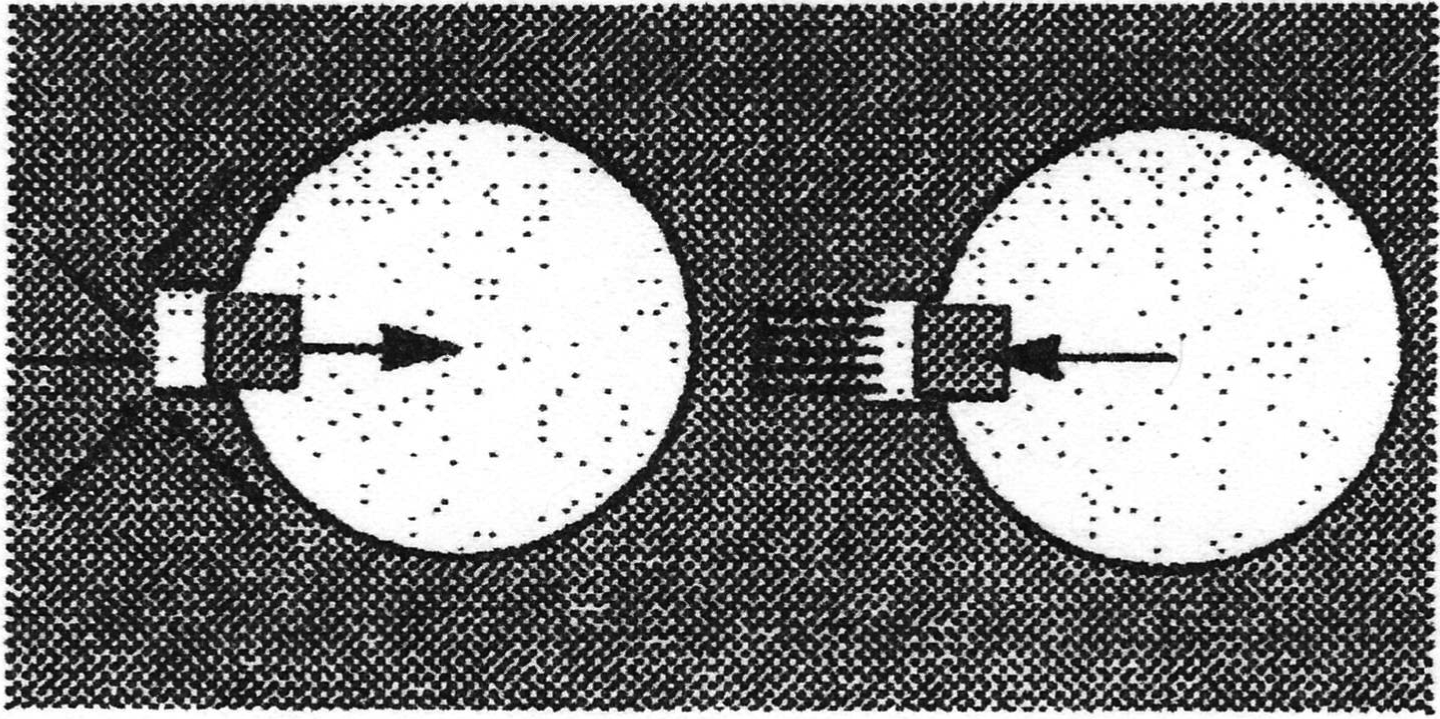Figure 3: Explanation of the force.

This object can be realized in an entertaining way with appropriate Christmas tree balls. The balls must have an open, cylindrical part. They can be fixed and balanced very easily with adhesive tape on a simple cross made of rectangular wood rods (figure 4). One can even combine two crosses with different ball sizes and opposite sense. These crosses have to be hung on a string with swivel snaps (as on fishing lines) so that they can rotate freely.


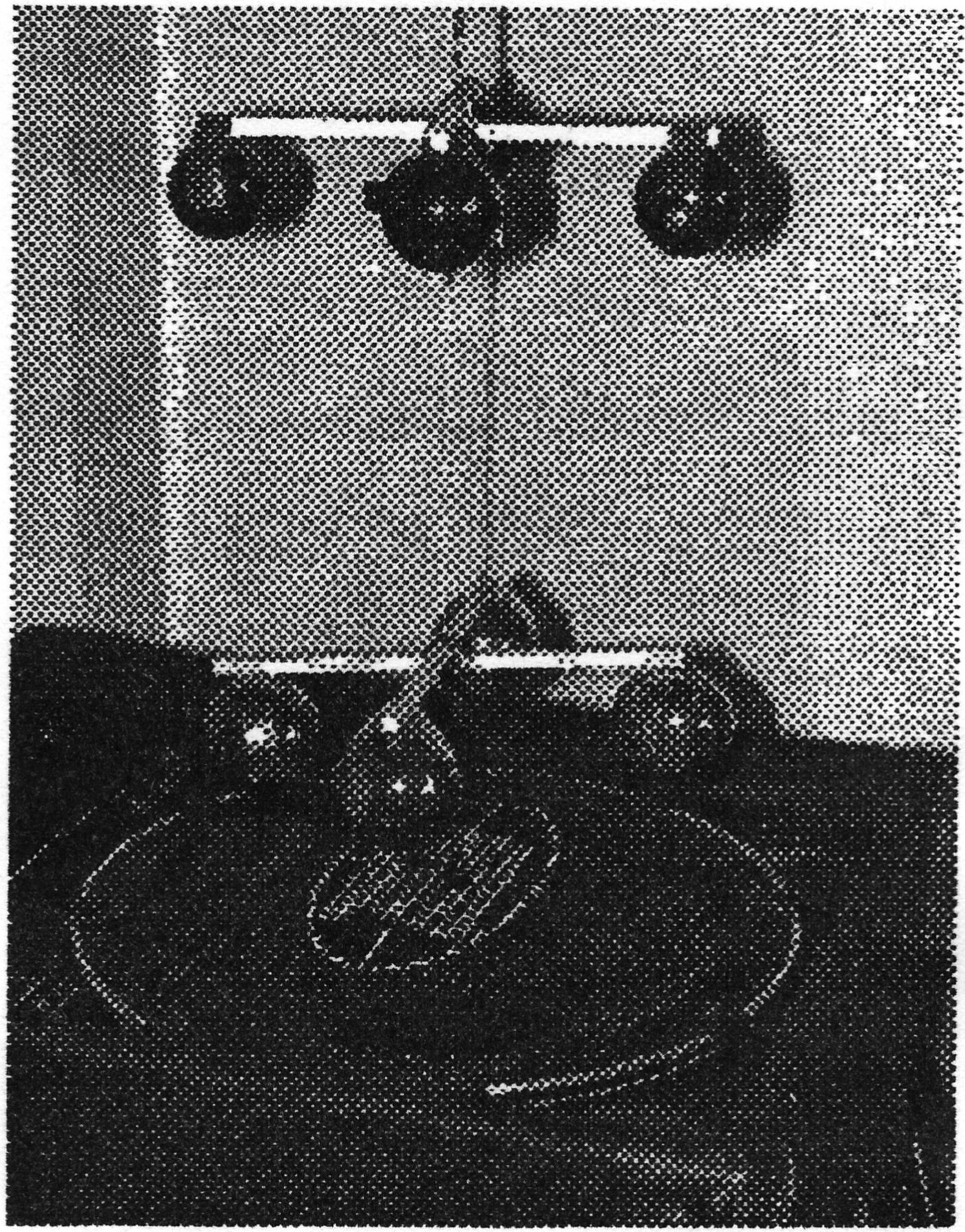Figure 4: The acoustical double wheel with Christmas tree balls. At the bottom the loudspeaker can be seen.

What you need now is a powerful amplifier with hundred-watt power, a good loudspeaker and a frequency generator. A computer speaker (about 50 W) connected with a soundcard works also but then the wheel rotates only slowly. The resonance frequency of the Christmas tree balls can be calculated only roughly with the formula because often the cylindrical part if not complete and not entirely cylindrical. Then one can find the best resonance frequency simply by experimenting. I was successful with Christmas tree balls with diameters of 4.4 cm (f_resonance ≈ 380 Hz) and 5 cm (f_resonance ≈ 580 Hz). The resonance frequency of a Christmas tree ball is relatively sharp.

It is also possible to operate the whole device with the right music which contains the resonance frequency. The power of music in contemporary discothèques is probably high enough. It is not recommended to use powerful Christmas music under the Christmas tree because this might disturb the meditative atmosphere of the festival of Christmas.

References

1. Mueller-Pouillet’s Lehrbuch der Physik und Meteorologie, Braunschweig, Vieweg 1906, page 790
2. Bergmann-Schaefer, Lehrbuch der Experimentalphysik Bd 1, Mechanik, Akustik, Waerme, Berlin 1975, page 523

---

Column Editor: Ernie McFarland, Physics Department, University of Guelph, Guelph, Ontario, N1G 2W1

Tags: Waves and Sound