Diana Hall, Bell High School, Nepean, Ontario

Here’s a really easy way to show students that the pressure at the end of an open-air column doesn’t change exactly at the physical end of the tube. It requires a motion sensor, a tube, and the right-sized insert for the tube. I happen to have a plexiglass tube into which a tub of play-doh fits just nicely.

Set up the following…



Now, while collecting position vs. time data, slowly draw the play-doh container out of the tube. When you get to the physical end of the tube, stop and hold a few seconds. Now slowly continue to draw the container out further.

What you should see is that the sound waves from the motion sensor reflect off the container as it is drawn out. Once the container passes the physical end of the tube, the waves continue to reflect off the container until it is a few millimetres past the end. Then the sound waves will stop “seeing” the container as they notice the change in air pressure outside the tube and now reflect off the open end.



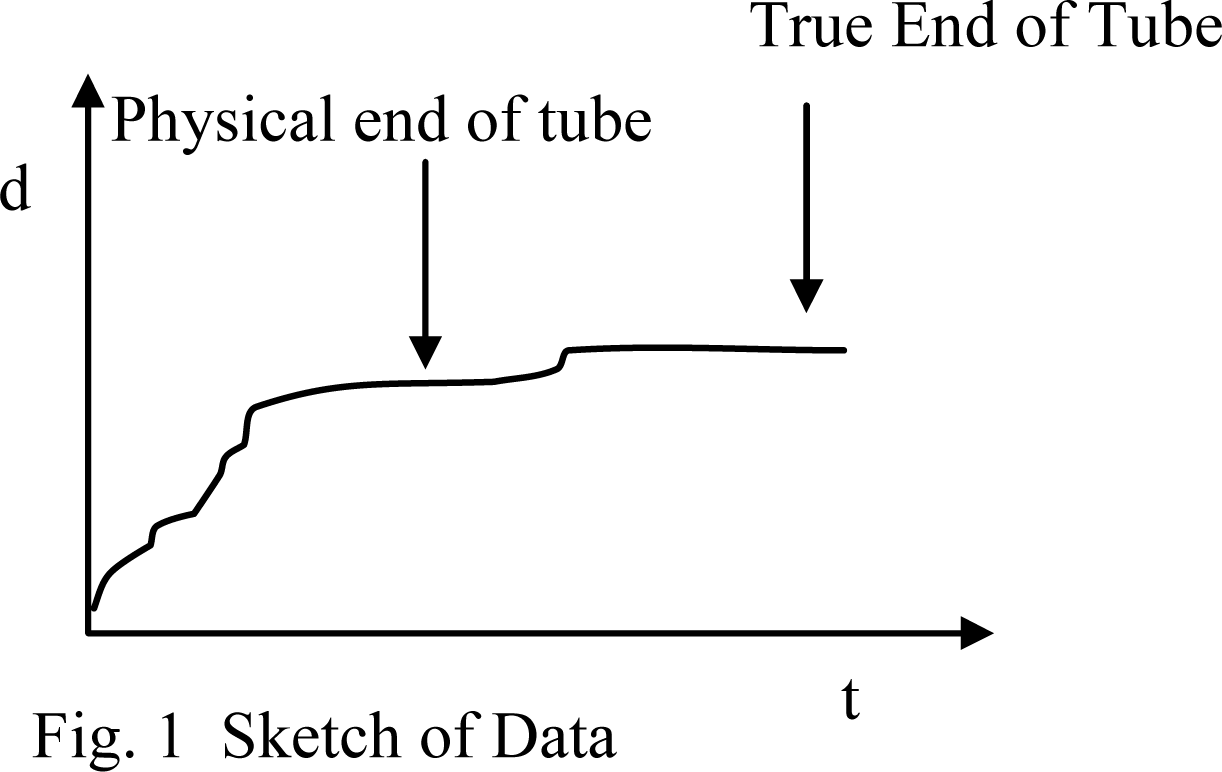
What’s the point? On the d-t graph you will see that the place where the sound waves reflect off the open end is beyond the physical end of the tube. Not by much mind, but nevertheless beyond. (See Fig. 1)



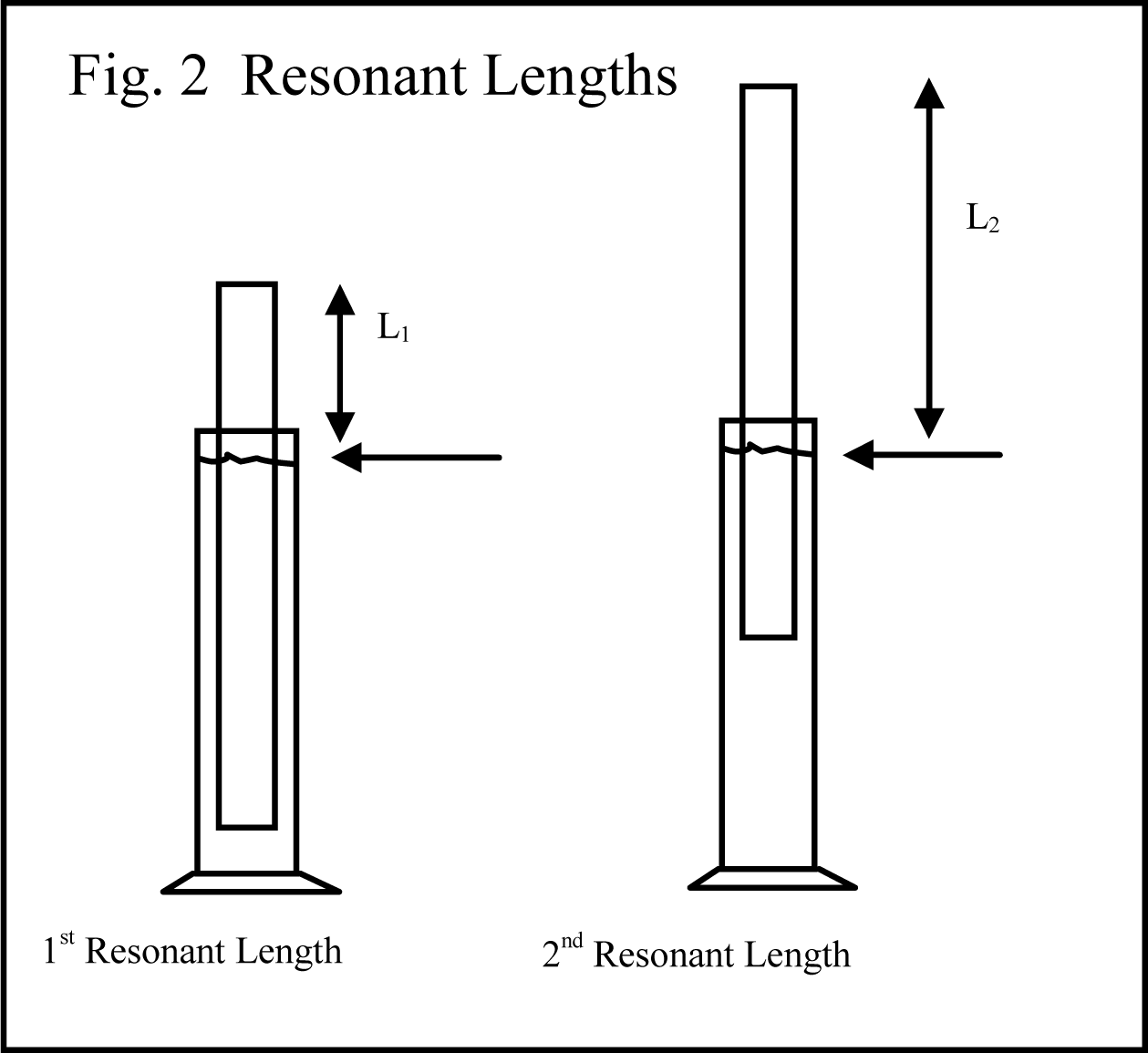
Who Cares? When conducting a lab to determine the speed of sound using a tuning fork of known frequency and an air column open at one end, you will get better results by finding two resonant lengths and subtracting them to find λ/2 (where λ is the wavelength), instead of using the first resonance length (for example) to give λ/4. Each of the two resonant lengths measured will have the same error at the open end, and subtracting the lengths to give ΔL = λ/2 also subtracts the open-end error. Fig. 2 shows two resonant lengths L2 and L1 measured from the top of water in the bottom container.

Student results for the speed of sound found by subtracting resonant lengths are typically within a few percent of the correct value, whereas results using a single resonant length can deviate by 10% or more from the correct value.

---

Column Editor: Ernie McFarland, Physics Department, University of Guelph, Guelph, Ontario, N1G 2W1

Tags: Waves and Sound